I have found a use for those unwanted compact discs that accumulate in the house. I got to thinking that the rim of a CD could represent a great circle on a sphere. Hmmmm.... How many ways can you lay great circles on a sphere in a symmetrical manner? How best to construct these figures? What do you call them?

I have done a few. It is fun to match up the printing such that it appears that the CDs interpenetrate. I have chosen to name them according to the straight-edged figure that would result by connecting the points of intersection of the CDs with straight lines instead of curves. Only figures that have an even number of edges meeting at each vertex are candidates for these balls.

Octahedron

 

Three CDs intersecting at right angles. This forms "corner reflectors" with the mirror-like CDs giving the illusion of transparency to the disks.

Cuboctahedron

 

Four CDs corresponding to the four 3-fold axes of a cube. This figure changes appearance greatly when viewed at different orientations.

Icosidodecahedron.

 

Six CDs representing the great circles corresponding to the six five-fold axes of the dodecahedron. The figure has twenty equilateral spherical triangles and twelve spherical pentagons.

Stellated Cube.

 

24 isosceles spherical triangles. The six CDs mark the great circles corresponding to the six two-fold axes of the cube.

Stellated Cuboctahedron.

8 equilateral spherical triangles and 24 isosceles spherical triangles. The seven CDs mark the great circles corresponding to the 3 four-fold axes of the cube plus the four 3-fold axes.

Other Models

By relaxing my requirement that the CDs lie on great circles, other figures are possible. The following ones are probably about as far as I intend to go with these models for a while.

Rhombicuboctahedron.

 

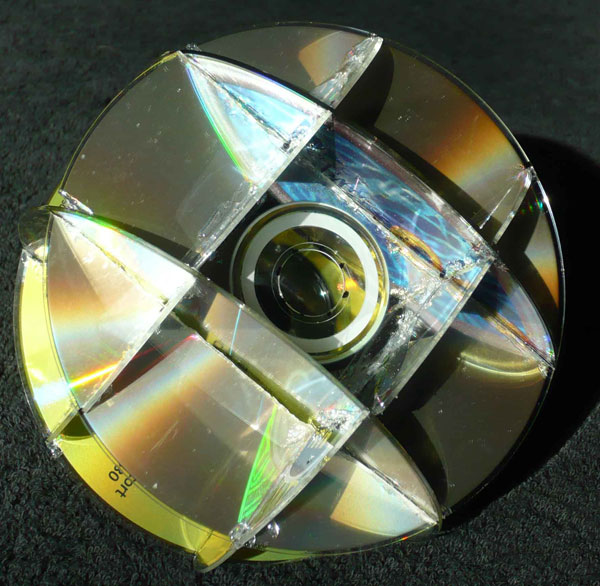
Six CDs here.

Rhombicosidodecahedron.

 

12 CDs. That's enough for me!

Please contact me with questions or comments.